Concept of speed:
Speed is an indication of how fast an object is moving. It is a scalar quantity that refers only to the magnitude, which means speed is something that’s measurable on a scale that shows how large it is.
The SI unit of speed m/s. The dimensional formula of speed is $[$$M^{0}LT^{-1}$$]$.
- Uniform speed: An object is said to be in uniform speed if it covers equal distances in equal intervals of time.
- Variable speed: An object covers equal distances in unequal intervals of time.
- Average speed: When an object moves with variable speed, the motion is expressed in average speed. It is defined as the total distance covered divided by the time taken to cover the distance.
Average speed = total distance covered/ time taken
d. Instantaneous speed: The speed of an object at any instant of time gives its
instantaneous speed.
Velocity:
Velocity is the rate at which an object changes its position in a specific direction. It is a vector quantity that refers to the direction the object has moved and measures distance of movement.
The SI unit of velocity m/s. The dimensional formula of velocity is $[$$M^{0}LT^{-1}$$]$.
- Uniform velocity: An object has a uniform velocity if it covers equal displacement in equal intervals of time. Uniform velocity means the magnitude, as well as direction, remains constant.
- Variable velocity: An object has a variable velocity if there is a change in its magnitude or direction or both.
- Average velocity: When an object moves with a variable velocity, the motion is expressed in average velocity. It is defined as the displacement covered divided by the time taken to cover the displacement.
Average velocity = displacement / time taken
d. Instantaneous velocity: The velocity of an object at any given instant of time gives its instantaneous velocity.
Acceleration:
Acceleration is defined as the rate of change of velocity with respect to time.
The average acceleration is defined as the change of velocity divided by the time interval.
Acceleration,
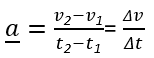
Where, $v_{2}$and $v_{1}$are the instantaneous velocities at time $t_{2}$and $t_{1}$.
The SI unit of acceleration is $m/s^{2}.$
Velocity-time graphs and acceleration:
Figure:2.a
Average acceleration: In the velocity-time graph, the slope of the line between the time interval $t_{1}$and $t_{2}$ gives the average value for the rate of change of velocity.
Instantaneous acceleration: In a velocity-time graph, the instantaneous acceleration is obtained by drawing the slope of the tangent on the v-t graph at any instant.
Positive, negative and zero acceleration:
Figure:2.b
It is observed from the graph that at the beginning, the velocity of an object is increasing, hence acceleration is positive.
In between 4s and 7s, velocity remains constant, hence acceleration is zero.
In between 7s and 10s, velocity is decreasing and hence acceleration is negative.
Determining displacement:
The area under the velocity-time graph represents the displacement of an object over a given time interval.
Figure:2.c
Displacement = area of ABEF
= area of triangle ABC + area of rectangle BCDE + area of triangle DEF
= ($\frac{1}{2}\times 4\times 8)$+ $(3\times 8)$+ ($\frac{1}{2}\times 3\times 8)$
= 16 + 24 + 12 = 52 m