Definition of Unit Cell
The smallest group of atoms which has the overall symmetry of a crystal, and from which the entire lattice can be built up by repetition in three dimensions is termed as Unit Cell. Crystalline solids exhibit a regular and repeating pattern of constituent particles. The diagrammatic representation of the three dimensional arrangement of constituent particles in a crystal, in which each particle is depicted as a point in space is known as crystal lattice.
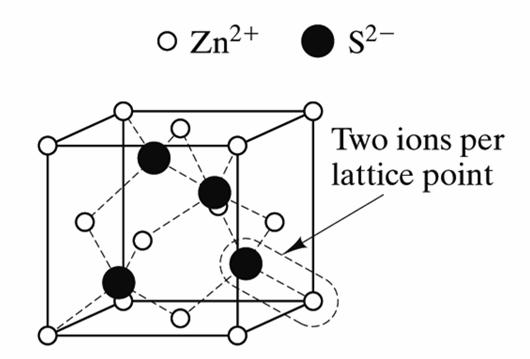
What is a Lattice?
A lattice is a framework, resembling a three-dimensional, periodic array of points, on which a crystal is built. In 1850, M. A. Bravais showed that identical points can be arranged spatially to produce 14 types of regular pattern. These 14 space lattices are known as Bravais lattices.
The crystal lattice of a solid can be described in terms of its unit cell. A crystal lattice is made up of a very large number of unit cells where every lattice point is occupied by one constituent particle. The unit cell can be seen as a three dimensional structure containing one or more atoms. We can determine the volume of this unit cell with the knowledge of the dimensions of the unit cell. For example: if we have a unit cell of edge “a”, the volume of the unit cell can be given as “a3”. Density of a unit cell is given as the ratio of mass and volume of unit cell. The mass of a unit cell is equal to the product of number of atoms in a unit cell and the mass of each atom in unit cell.
Mass of unit cell = number of atoms in unit cell × mass of each atom = z × m
Where, z = number of atoms in unit cell,
m = Mass of each atom
Mass of an atom can be given with the help of Avogadro number and molar mass as:
MNA
Where, M = molar mass
NA = Avogadro’s number
Volume of unit cell, V = a3
=> Density of unit cell = mass of unit cell volume of unit cell
=> Density of unit cell = mV = z × ma3 = z × Ma3 × NA
Thus, with the knowledge of number of atoms in a unit cell, edge length and molar mass we can determine the density of a unit cell.
A general expression for density of unit cell for various cases has been derived below:
Primitive unit cell: In a primitive unit cell, number of atoms in a unit cell, z is equal to one. Hence density is given as:
Density of unit cell = 1 × Ma3 × NA
Body-centered cubic unit cell: In body-centered cubic unit cell, number of atoms in a unit cell, z is equal to two. Hence density is given as:
Density of unit cell = 2 × Ma3 × NA
Face-centered cubic unit cell: In face-centered cubic unit cell, number of atoms in a unit cell, z is equal to four. Hence density of unit cell is given as:
Density of unit cell = 4 × Ma3 × NA