Vapor pressure is the pressure applied by the vapor when a vapor is in “equilibrium state” with solid or liquid form, or both, of the identical substance – that is when conditions are like this that the substance can be present in both or exist in all three phases.
Vapor Pressure refers to the measure of the tendency of the substance to transform in the vapor or gaseous state, and it accelerates with temperature.
At the temperature at which the vapor pressure acting on liquid’s surface becomes equal to the pressure applied by the surrounding atmosphere, it is called the boiling point of the liquid.
Equilibrium Vapor Pressure
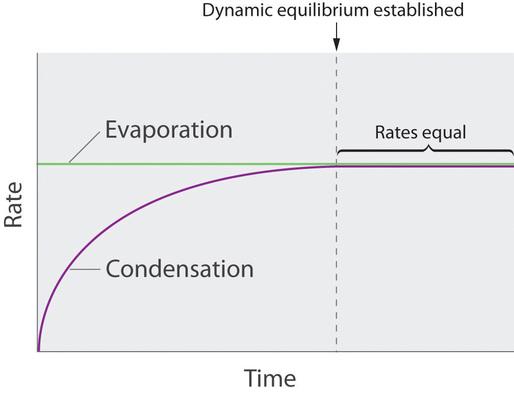
When you talk of two opposite process like condensation and evaporation that takes place at the same rate and therefore produces no pure or clear change in the system and comprises a “dynamic equilibrium.” if, in any condition, the liquid is confined in a chamber, then continuously the molecules will condense and evaporate but the liquid and vapor amount don’t change w.r.t time. In dynamic equilibrium, the pressure applied by the vapor with the liquid is known as the liquid’s “equilibrium vapor pressure”.
If the liquid is in the open container, nonetheless mostly all the molecules that break out into the vapor phase which will not collide with liquid’s surface and will come back to the liquid phase. Rather, they will diffuse via the gas phase at a far distance from the container, and the equilibrium will never be acknowledged. Under such conditions, the liquid will continue the evaporation process until it “disappears”.
Speed or the rate of motion with which it occurs is totally dependent on temperature and liquid’s vapor pressure. Comparatively, volatile liquids have higher vapor pressures and they evaporate much quickly, whereas, the non-volatile liquids consist of low vapor pressures and they tend to evaporate very slowly.
As a common guideline, the separating line between non-volatile and volatile liquids is not definite, so, we can claim that substances having greater vapor pressures than water are comparatively volatile, whereas, substances with lower vapor pressures than water are comparatively non-volatile.
Therefore, acetone, diethyl ether or ethyl ether is volatile and ethylene, motor oil, and glycol are non-volatile.
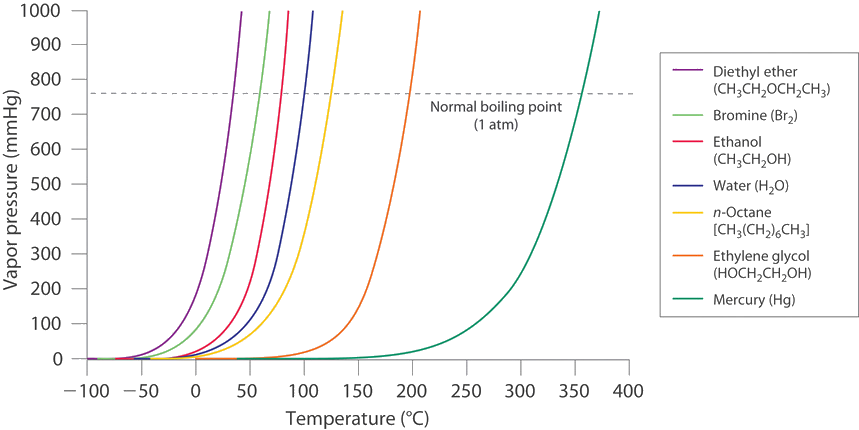
Fig 1. The Vapor Pressures of different Liquids as a Function temperature. The particular point at which the curve of the vapor pressure crosses the Pressure (P) = 1 atm line (dashed or dotted line) is the normal liquid’s boiling point.
The vapor pressure or equilibrium vapor pressure of the substance at a specific temperature is the characteristic of a material such as its boiling point, molecular mass, and the melting point. It’s not dependent on the quantity of liquid considering at least a small amount of liquid exists in equilibrium with a vapor. Equilibrium vapor pressure, however, relies very strongly on intermolecular forces and temperature present, as shown in the figure for other substances.
Figure 1. shows the exponential rise in vapor pressure with temperature increase in and allowing us to have the benefit of natural logarithms to signify the nonlinear relationship as the linear one.
H in (P) = −ΔHvap/R (1/T) +C —————————– Eq. 1
Where,
- Ln P = natural logarithm of the vapor pressure,
- ΔHvap = Enthalpy of vaporization,
- R = universal gas constant [8.314 J/(mol•K)],
- T = temperature in kelvins
- C = y-intercept, which is constant for any given line.
P Vs the inverse of the absolute temperature i.e. (1/T) is a straight line w.r.t slope of −ΔHvap/R. Eq. 1 is referred to as the Clausius–Clapeyron equation can be used for the calculation of ΔHvap of a fluid from its measured vapor pressure at two or more than two temperatures and put P and T values for these points into Equation 2
ln (P1P2) = −ΔHvap/R(1/T1−1/T2) ………………….Eq. 2
Vice-versa if we know about P1, vapor pressure and ΔHvap at any temperature, T1, we can use equation 2 for the calculation of P2 (vapor pressure) at any other T2 temperature as shown in equation 1.